Slush flows
Slush flows
Contents
What are slush flows
Project team
Summary of the research plan and objectives
What are slush flows ?
Slush stream is the expression for the general phenomena of the movement of water
saturated snow downhill. Slush streams are confined to channels and are occuring on gentle
slopes of less than 20°. They can therefore be well distinguished from avalanches, which
are normally initiated on slopes of more than 20°inclination.
The magnitude of slush streams show a wide range. The high energetic variant of slush streams, called slush torrents, show extreme magnitudes with velocities higher than 20 m/s and discharge values up to some thousands of m^3/s. Slush streams have been reported from arctic and subpolar regions, but there are also indications of their occurrence in the Swiss Alps.
Project team
In december 1995, a research project with the title "Investigation of Physical
Principles and Modelling Prerequisits of Slush Streams" started at the MCR Lab. This
project is financed by the Swiss National Found (SNF). The project team consists of :
Project coordinator : Dr. D Scherer and Prof. Dr. E. Parlow
Scientist : Dipl.- Geogr. M Gempeler
Summary of the research plan and
objectives
The results from three arctic spring and summer expeditions to Liefdefjorden in
NW-Spitsbergen have led to a new understanding and definition of slush streams. These
processes, which sometimes have been mismatched with wet snow avalanches, have been
studied in detail by the research groups from Basel and Heidelberg during an international
project (SPE'90-92). Due to the special climatic conditions and their related phenomena,
slush streams, which are of great importance for sediment and water budgets, are mostly
observed at high latitudes. In these regions, snowmelt mainly occurs during one distinct
period in later spring and reveals strong similarities between different drainage basins,
compared to the more complex spatial and temporal patterns in alpine regions at lower
latitudes.
A new physical concept for the release mechanism of slush streams is based on the
assumption that the development of a hydrostatic pressure gradient due to the generation
of an inclined water table in the snow cover is mainly responsible for slush stream
initiation. The critical factor for slush stream release is the snow cover state, since
the hydrostatic pressure gradient is balanced by the shear stability of the snow matrix as
long as the pressure gradient does not exceed a certain threshold. Therefore, a detailed
analysis of all available data is necessary to get an improved understanding of these
highly dynamic phenomena. These data sets comprise of field data, observations, digital
terrain information layers, but also of satellite imagery from Landsat-TM and ERS-1. Slush
stream relevant information, already and completely available from NW-Spitsbergen, are
complemented by new field data from an investigation in the Kärkevagge in North-Sweden,
which took place in May and June 1995 as an international collaboration.
The second objective of this project is to examine and evaluate the model prerequisites
for the development of a physically based and fully distributed snowmelt model. To
simulate the dynamic aspects of slush streams, it is obvious that such a model demands
distributed information of all relevant energy budget components such as net radiation,
sensible and latent heat fluxes to compute the snowmelt rate. In addition, a distribution
of snow cover state variables as snow depth, density, shear stability and others is also
prerequisite for such a model. Therefore, this project focuses on thematic and
methodological problems that have to be solved before a dynamic model can be constructed
to simulate slush streams. This proposed investigation will be a contribution both to the
question of the physical principles of slush streams and to the problem of modelling such
highly dynamic processes of snowmelt at high latitudes.
Research strategy
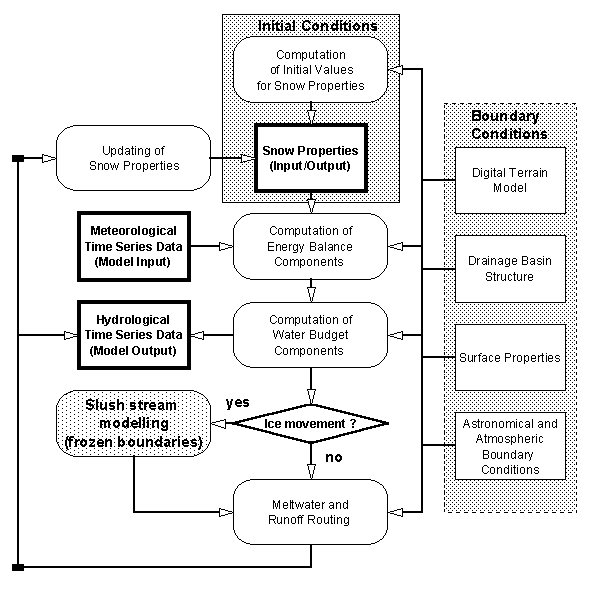
The following figure illustrates the general research strategy of this project:

The following structural parameters and state variables or processes are considered by
this investigation (mentioned are only the most important ones).
Relief: Elevation (usually in m a.s.l.), aspect, inclination, curvature (total,
horizontal, vertical), horizon angles (for 24 azimuth angles), normalized sky view factor,
surface ratio, relief energy.
Drainage basin: Total area, drainage basin assignment, divide, location and elevation of
the outlet, partial drainage basin area, distance to the outlet, channel network,
channelled runoff, overland flow.
Snow cover: Snow depth, density and temperature distribution, snow water equivalent, ice,
water and air content, water vapour pressure, crystal radii, shear stability, further
physical parameters (e.g. heat capacity and conductivity, solar reflectance, emittance),
settling and compaction, phase transitions, ice and water transports.
Surface and sublayer: Surface type (e.g. water bodies, glaciers, rocks, soils), vegetation
(e.g. type, coverage, biomass, height), physical parameters of the sublayer, permafrost
conditions (e.g. temperature, ice content, active layer depth), ice and water content of
soils, energy and water transports in the sublayer.
Climate: Long-term monthly means of the most important variables (e.g. air temperature,
humidity, precipitation).
Meteorology: General (synoptic) weather conditions (e.g. air pressure, height, temperature
and wind at the 500 and 200 hPa level) on a daily basis, daily or hourly means of further
meteorological variables, vertical profiles of air pressure, temperature and humidity in
the free atmosphere, coupling of the planetary boundary layer to the free atmosphere,
radiation budget and energy balance at the actual surface.
Modelling
Snow is regarded as a system of four components. Ice, water and water vapour represent the
three phases of H2O, while dry air is sharing its volume with water vapour, and therefore
will be taken together as gaseous component by some of the modules. First of all, the
sublayer comprises a static matrix, and its pore volume is filled by ice, water and wet
air, in the case of soils and rocks.
Most parts of a future model are expected to be solved on a spatial grid, since all
distributed variables are stored as two-dimensional arrays. Topology is provided by the
DEM; the third dimension results from the vertical coupling of the two-dimensional arrays.
The channelled drainage system is derived from a grid-based digital relief analysis, and
afterwards transformed into a vector representation, which empowers the simulation of
channelled runoff by means of a network algorithm. The drainage channel network is coupled
to the model grid to allow lateral transports of water into or out of the channel system.
This approach provides the coupling of two process systems of two different time scales
without wasting computer time by avoiding the higher frequency in the whole model.
Otherwise, the high temporal resolution would be necessary for a finite element algorithm
in the module of fluvial dynamics simulation.
The principle idea of a model set-up is given by the following flow chart: